Capillary action – how contact angle and surface tension are related?

Capillary action, also called capillary filling or wicking, means the spontaneous flow of liquid to fill small capillaries or pores. A famous example is that of capillary rise: when a glass capillary with a small diameter is placed in water, the level of water inside the capillary rises above the level of water outside the tube. This phenomenon is driven by surface tension forces which is why the smaller the capillary, the higher the water level will rise.

Capillary action does not only apply to water but is equally applicable to any liquid: water, oils, organic solvents, biological fluids, etc. Capillary action has a major role in many natural, industrial and commercial processes. Examples of natural processes include the transport of water from the roots of trees to treetops and the movement of water in aquifers. Examples of industrial and commercial processes include oil extraction and lateral flow home diagnostic tests.

Capillary filling is spontaneous when the free energy change is negative

Capillary action is caused by the minimization of the surface free energy. Young’s equation describes the relation of the contact angle θ and the surface (and interfacial) free energies of the system as:

γ_lv *cos(θ) = γ_sv - γ_sl ,

where and γ_lv, γ_sv, and γ_sl are free energies of the liquid-vapor, the solid-vapor, and the solid-liquid interfaces.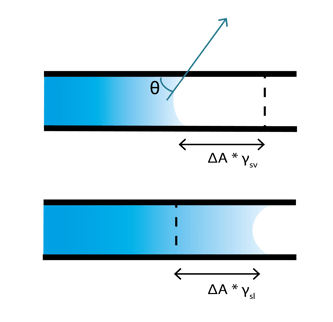

When a new section of a capillary is wetted, some amount of solid-vapor surface area (ΔA) is lost from the previously dry channel and simultaneously the same amount of new solid-liquid interfacial area is created. The free energy change (ΔE) associated with this is

ΔE = (γ_sl - γ_sv)*ΔA .

To link the free energy change to the contact angle, we apply Young’s equation to get

ΔE = - γ_lv *cos(θ)*ΔA .

For a hydrophilic surface, the contact angle θ is < 90° which means that the free energy change of wetting the capillary is negative i.e. the filling happens spontaneously.

Capillary action can also be to understood in terms of capillary pressure

Capillary action can also be understood in terms of capillary pressure. There’s a pressure difference, called the Laplace pressure, across all curved liquid interfaces caused by unbalanced forces between the liquid molecules at the interface. For the case of a pore with a circular cross-section (radius r) the capillary pressure is given by

P_cap = 2 γ_lv *cos(θ) / r.

From this, we see that the lower the contact angle (the more hydrophilic the pore) the higher the capillary pressure and thus the the stronger the capillary action. We also see that the smaller the pore is the stronger the capillary action. For this reason, the capillary filling is not a major factor in macroscopic systems where the characteristic dimension is much larger than 1 mm. The effect of surface tension on capillary pressure is more complicated: on one hand, the capillary pressure is directly proportional to the surface tension. On the other hand, high surface tension liquids typically also have higher contact angles, which lower the capillary pressure. It should be emphasized that the two ways to understand the capillary action presented above (free energy change or capillary pressure) are two descriptions of the same physical phenomenon and not two different phenomena.

With this information, it’s possible to predict how liquids will behave in applications, such as a lateral flow medical diagnostic test, based on the dimensions, the contact angle and the surface tension of a liquid. Such a test is used to analyze samples of e.g. blood or urine whose surface tensions are lower than water but still reasonably high. Therefore, the materials utilized in such tests need to be very hydrophilic for reliable capillary filling, for example, glass, hydrophilic paper or hydrophilized polymers. On the other hand, in applications where the liquid transported is e.g. ethanol (with much lower contact angle towards the polymer), even inherently hydrophobic polymers can be utilized.